PID controller

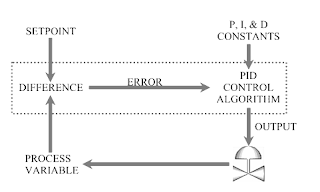
The P stands for proportional control, I for integral control and D for derivative control. This is also what is called a three term controller.

The basic function of a controller is to execute an algorithm (electronic controller) based on the control engineer's input (tuning constants), the operators desired operating value (setpoint) and the current plant process value. In most cases, the requirement is for the controller to act so that the process value is as close to the setpoint as possible. In a basic process control loop, the control engineer utilises the PID algorithms to achieve this.

The PID control algorithm is used for the control of almost all loops in the process industries, and is also the basis for many advanced control algorithms and strategies. In order for control loops to work properly, the PID loop must be properly tuned. Standard methods for tuning loops and criteria for judging the loop tuning have been used for many years, but should be reevaluated for use on modern digital control systems.

While the basic algorithm has been unchanged for many years and is used in all distributed control systems, the actual digital implementation of the algorithm has changed and differs from one system to another.

How a PID Controller Works

The PID controllers job is to maintain the output at a level so that there is no difference (error) between the process variable (PV) and the setpoint (SP).

In the diagram shown above the valve could be controlling the gas going to a heater, the chilling of a cooler, the pressure in a pipe, the flow through a pipe, the level in a tank, or any other process control system.

What the PID controller is looking at is the difference (or "error") between the PV and the SP. It looks at the absolute error and the rate of change of error. Absolute error means -- is there a big difference in the PV and SP or a little difference? Rate of change of error means -- is the difference between the PV or SP getting smaller or larger as time goes on.

When there is a "process upset", meaning, when the process variable or the setpoint quickly changes - the PID controller has to quickly change the output to get the process variable back equal to the setpoint. If you have a walk-in cooler with a PID controller and someone opens the door and walks in, the temperature (process variable) could rise very quickly. Therefore the PID controller has to increase the cooling (output) to compensate for this rise in temperature.

Once the PID controller has the process variable equal to the setpoint, a good PID controller will not vary the output. You want the output to be very steady (not changing). If the valve (motor, or other control element) are constantly changing, instead of maintaining a constant value, this could case more wear on the control element.

So there are these two contradictory goals. Fast response (fast change in output) when there is a "process upset", but slow response (steady output) when the PV is close to the setpoint.

Note that the output often goes past (over shoots) the steady-state output to get the process back to the setpoint. For example, a cooler may normally have it's cooling valve open 34% to maintain zero degrees (after the cooler has been closed up and the temperature settled down). If someone opens the cooler, walks in, walks around to find something, then walks back out, and then closes the cooler door -- the PID controller is freaking out because the temperature may have raised 20 degrees! So it may crank the cooling valve open to 50, 75, or even 100 percent -- to hurry up and cool the cooler back down -- before slowly closing the cooling valve back down to 34 percent.

proportional & derivative control.pdf

Supervisory Contorl & Data Aquisition

Following we describe the SCADA systems in terms of their architecture, their interface to the process hardware, the functionality and the application development facilities they provide.

SCADA systems have made substantial progress over the recent years in terms of functionality, scalability, performance and openness such that they are an alternative to in house development even for very demanding and complex control systems.

What does SCADA mean?
SCADA stands for Supervisory Control And Data Acquisition. As the name indicates, it is not a full control system, but rather focuses on the supervisory level. As such, it is a purely software package that is positioned on top of hardware to which it is interfaced, in general via Programmable Logic Controllers (PLC's), or other commercial hardware modules.

SCADA systems are used not only in industrial processes: e.g. steel making, power generation (conventional and nuclear) and distribution, chemistry, but also in some experimental facilities such as nuclear fusion. The size of such plants range from a few 1000 to several 10 thousands input/output (I/O) channels. However, SCADA systems evolve rapidly and are now penetrating the market of plants with a number of I/O channels of several 100 thousands I/O's

SCADA systems used to run on DOS, VMS and UNIX; in recent years all SCADA vendors have moved to NT, Windows XP, Windows Server 2003 and some also to Linux.

1. Architecture

This section describes the common features of the SCADA products.

Hardware Architecture
One distinguishes two basic layers in a SCADA system: the "client layer" which caters for the man machine interaction and the "data server layer" which handles most of the process data control activities. The data servers communicate with devices in the field through process controllers. Process controllers, e.g. PLC's, are connected to the data servers either directly or via networks or fieldbuses that are proprietary (e.g. Siemens H1), or non-proprietary (e.g. Profibus). Data servers are connected to each other and to client stations via an Ethernet LAN.

Software Architecture
The products are multi-tasking and are based upon a real-time database (RTDB) located in one or more servers. Servers are responsible for data acquisition and handling (e.g. polling controllers, alarm checking, calculations, logging and archiving) on a set of parameters, typically those they are connected to.

However, it is possible to have dedicated servers for particular tasks, e.g. historian, datalogger, alarm handler. The figure above shows a generic SCADA software architecture.

Communications

Internal Communication:
Server-client and server-server communication is in general on a publish-subscribe and event-driven basis and uses a TCP/IP protocol, i.e., a client application subscribes to a parameter which is owned by a particular server application and only changes to that parameter are then communicated to the client application.

Access to Devices
The data servers poll the controllers at a user defined polling rate. The polling rate may be different for different parameters. The controllers pass the requested parameters to the data servers. Time stamping of the process parameters is typically performed in the controllers and this time-stamp is taken over by the data server. If the controller and communication protocol used support unsolicited data transfer then the products will support this too.

The products provide communication drivers for most of the common PLC's and widely used field-buses, e.g., Modbus. Some of the drivers are based on third party products (e.g., Applicom cards) and therefore have additional cost associated with them. VME on the other hand is generally not supported.

A single data server can support multiple communications protocols: it can generally support as many such protocols as it has slots for interface cards.

The effort required to develop new drivers is typically in the range of 2-6 weeks depending on the complexity and similarity with existing drivers, and a driver development toolkit is provided for this.

Interfacing

Application Interfaces / Openness:
The provision of OPC client functionality for SCADA to access devices in an open and standard manner is developing. There still seems to be a lack of devices/controllers, which provide OPC server software, but this improves rapidly as most of the producers of controllers are actively involved in the development of this standard.

The products also provide an Open Data Base Connectivity (ODBC) interface to the data in the archive/logs, but not to the configuration database, an ASCII import/export facility for configuration data, a library of APIs supporting C, C++, and Visual Basic (VB) to access data in the RTDB, logs and archive. The API often does not provide access to the product's internal features such as alarm handling, reporting, trending, etc.

The PC products provide support for the Microsoft standards such as Dynamic Data Exchange (DDE) which allows e.g. to visualize data dynamically in an EXCEL spreadsheet, Dynamic Link Library (DLL) and Object Linking and Embedding (OLE).

Database
The configuration data are stored in a database that is logically centralised but physically distributed and that is generally of a proprietary format.

For performance reasons, the RTDB resides in the memory of the servers and is also of proprietary format.

The archive and logging format is usually also proprietary for performance reasons, but some products do support logging to a Relational Data Base Management System (RDBMS) at a slower rate either directly or via an ODBC interface.

Scalability

Scalability is understood as the possibility to extend the SCADA based control system by adding more process variables, more specialized servers (e.g. for alarm handling) or more clients. The products achieve scalability by having multiple data servers connected to multiple controllers. Each data server has its own configuration database and RTDB and is responsible for the handling of a sub-set of the process variables (acquisition, alarm handling, archiving).

Redundancy
The products often have built in software redundancy at a server level, which is normally transparent to the user. Many of the products also provide more complete redundancy solutions if required.

2. Functionality

Access Control
Users are allocated to groups, which have defined read/write access privileges to the process parameters in the system and often also to specific product functionality.

MMI
The products support multiple screens, which can contain combinations of synoptic diagrams and text.

They also support the concept of a "generic" graphical object with links to process variables. These objects can be "dragged and dropped" from a library and included into a synoptic diagram.

Most of the SCADA products that were evaluated decompose the process in "atomic" parameters (e.g. a power supply current, its maximum value, its on/off status, etc.) to which a Tag-name is associated. The Tag-names used to link graphical objects to devices can be edited as required. The products include a library of standard graphical symbols, many of which would however not be applicable to the type of applications encountered in the experimental physics community.

Standard windows editing facilities are provided: zooming, re-sizing, scrolling... On-line configuration and customization of the MMI is possible for users with the appropriate privileges. Links can be created between display pages to navigate from one view to another.

Trending
The products all provide trending facilities and one can summarize the common capabilities as follows:

● the parameters to be trended in a specific chart can be predefined or defined on-line
● a chart may contain more than 8 trended parameters or pens and an unlimited number of charts can be displayed (restricted only by the readability)
● real-time and historical trending are possible, although generally not in the same chart
● historical trending is possible for any archived parameter
● zooming and scrolling functions are provided
● parameter values at the cursor position can be displayed

The trending feature is either provided as a separate module or as a graphical object (ActiveX), which can then be embedded into a synoptic display. XY and other statistical analysis plots are generally not provided.

Alarm Handling
Alarm handling is based on limit and status checking and performed in the data servers. More complicated expressions (using arithmetic or logical expressions) can be developed by creating derived parameters on which status or limit checking is then performed. The alarms are logically handled centrally, i.e., the information only exists in one place and all users see the same status (e.g., the acknowledgement), and multiple alarm priority levels (in general many more than 3 such levels) are supported.

It is generally possible to group alarms and to handle these as an entity (typically filtering on group or acknowledgement of all alarms in a group). Furthermore, it is possible to suppress alarms either individually or as a complete group. The filtering of alarms seen on the alarm page or when viewing the alarm log is also possible at least on priority, time and group. However, relationships between alarms cannot generally be defined in a straightforward manner. E-mails can be generated or predefined actions automatically executed in response to alarm conditions.

Logging/Archiving
The terms logging and archiving are often used to describe the same facility. However, logging can be thought of as medium-term storage of data on disk, whereas archiving is long-term storage of data either on disk or on another permanent storage medium. Logging is typically performed on a cyclic basis, i.e., once a certain file size, time period or number of points is reached the data is overwritten. Logging of data can be performed at a set frequency, or only initiated if the value changes or when a specific predefined event occurs. Logged data can be transferred to an archive once the log is full. The logged data is time-stamped and can be filtered when viewed by a user. The logging of user actions is in general performed together with either a user ID or station ID. There is often also a VCR facility to play back archived data.

Report Generation
One can produce reports using SQL type queries to the archive, RTDB or logs. Although it is sometimes possible to embed EXCEL charts in the report, a "cut and paste" capability is in general not provided. Facilities exist to be able to automatically generate, print and archive reports.

Automation
The majority of the products allow actions to be automatically triggered by events. A scripting language provided by the SCADA products allows these actions to be defined. In general, one can load a particular display, send an Email, run a user defined application or script and write to the RTDB.

The concept of recipes is supported, whereby a particular system configuration can be saved to a file and then re-loaded at a later date.

Sequencing is also supported whereby, as the name indicates, it is possible to execute a more complex sequence of actions on one or more devices. Sequences may also react to external events.

3. Application Development

Configuration
The development of the applications is typically done in two stages. First the process parameters and associated information (e.g. relating to alarm conditions) are defined through some sort of parameter definition template and then the graphics, including trending and alarm displays are developed, and linked where appropriate to the process parameters. The products also provide an ASCII Export/Import facility for the configuration data (parameter definitions), which enables large numbers of parameters to be configured in a more efficient manner using an external editor such as Excel and then importing the data into the configuration database.

However, many of the PC tools now have a Windows Explorer type development studio. The developer then works with a number of folders, which each contains a different aspect of the configuration, including the graphics.

The facilities provided by the products for configuring very large numbers of parameters are not very strong. However, this has not really been an issue so far for most of the products to-date, as large applications are typically about 50k I/O points and database population from within an ASCII editor such as Excel is still a workable option.

Online modifications to the configuration database and the graphics are generally possible with the appropriate level of privileges.

Development Tools
The following development tools are provided as standard:
● Graphics Editor, with standard drawing facilities including freehand, lines, squares circles, etc. It is possible to import pictures in many formats as well as using predefined symbols including e.g. trending charts, etc. A library of generic symbols is provided that can be linked dynamically to variables and animated as they change. It is also possible to create links between views so as to ease navigation at run-time.
● Database Configuration Tool (usually through parameter templates). It is in general possible to export data in ASCII files so as to be edited through an ASCII editor or Excel.
● Scripting Language
● Application Program Interface (API) supporting C, C++, VB
● Driver Development Toolkit to develop drivers for hardware that is not supported by the SCADA product.

Object Handling
The products in general have the concept of graphical object classes, which support inheritance. In addition, some of the products have the concept of an object within the configuration database. In general the products do not handle objects, but rather handle individual parameters, e.g., alarms are defined for parameters, logging is performed on parameters, and control actions are performed on parameters. The support of objects is therefore fairly superficial.


4. Evolution

SCADA vendors release one major version and one to two additional minor versions once per year. These products evolve thus very rapidly so as to take advantage of new market opportunities, to meet new requirements of their customers and to take advantage of new technologies.

As was already mentioned, most of the SCADA products that were evaluated decompose the process in "atomic" parameters to which a Tag-name is associated. This is impractical in the case of very large processes when very large sets of Tags need to be configured. As the industrial applications are increasing in size, new SCADA versions are now being designed to handle devices and even entire systems as full entities (classes) that encapsulate all their specific attributes and functionality. In addition, they will also support multi-team development.

As far as new technologies are concerned, the SCADA products are now adopting:
● Web technology, ActiveX, Java, etc.
● OPC as a means for communicating internally between the client and server modules. It should thus be possible to connect OPC compliant third party modules to that SCADA product.


5. Engineering

Whilst one should rightly anticipate significant development and maintenance savings by adopting a SCADA product for the implementation of a control system, it does not mean a "no effort" operation. The need for proper engineering can not be sufficiently emphasized to reduce development effort and to reach a system that complies with the requirements, that is economical in development and maintenance and that is reliable and robust. Examples of engineering activities specific to the use of a SCADA system are the definition of:

● A library of objects (PLC, device, subsystem) complete with standard object behavior (script, sequences, ...), graphical interface and associated scripts for animation
● Templates for different types of "panels", e.g. alarms
● Instructions on how to control e.g. a device ...
● A mechanism to prevent conflicting controls (if not provided with the SCADA)
● Alarm levels, behavior to be adopted in case of specific alarms.

links:- scada.pdf

Programmable Logic Controller

A PROGRAMMABLE LOGIC CONTROLLER (PLC) is an industrial computer control system that continuously monitors the state of input devices and makes decisions based upon a custom program, to control the state of devices connected as outputs.Almost any production line, machine function or process can be automated using a PLC. The speed and accuracy of the operation can be greatly enhanced using this type of control system. But the biggest benefit in using a PLC is the ability to change and replicate the operation or process while collecting and communicating vital information.

>> free PLC tutorial <<

Useful links for PLC information:- PLC pimer(252k pdf file)

for the beginners in PLC.

programming & documentation pads(ALL) (720k pdf file)

These useful PLC listing pads allow you to implement better

PLC design implementations and keep an orderly

documentation system. Select a PDF with all listing and sample pads or individual listings with sample.

PLC Program listing(184k pdf file)

Input/Output Listing(200K PDF file)

Register-Word/Internal Output Usage Listing (196K PDF file)

PLC Automation Project Notes (168K PDF file)

PLC Glossary of Terms (92K PDF file)

Distributed Control System

Distributed Control System (DCS)

The DCS is a control system which collects the data from the field and decides what to do with them. Data from the field can either be stored for future reference, used for simple process control, use in conjunction with data from another part of the plant for advanced control strategies.What must be in the DCS for it to be able to do so much?

Operator Console
These are like the monitors of our computers. They provide us with the feedback of what they are doing in the plant as well as the command we issue to the control system. These are also the places where operators issue commands to the field instruments.
Engineering StationThese are stations for engineers to configure the system and also to implement control algorithms.

History Module

This is like the harddisk of our PCs. They store the configurations of the DCS as well as the configurations of all the points in the plant. They also store the graphic files that are shown in the console and in most systems these days they are able to store some plant operating data.

Data Historian

These are usually extra pieces of software that are dedicated to store process variables, set points and output values. They are usually of higher scanning rates than that available in the history module.

Control Modules

These are like the brains of the DCS. Specially customized blocks are found here. These are customized to do control functions like PID control, ratio control, simple arithmetic and dynamic compensation. These days, advanced control features can also be found in them.I/OThese manage the input and output of the DCS. Input and output can be digital or analogues. Digital I/Os are those like on/off, start/stop signals. Most of the process measurements and controller outputs are considered analogue. These are the points where the field instruments are hard-wired to.
All above mentioned elements are connected by using a network, nowadays very often used is Ethernet.The practical and technological boundaries between a Distributed Control System DCS, Programmable Logic Controller PLC and Personal Computer PC control are blurring. Systems traditionally associated with process control are being used in discrete applications. Likewise, traditionally discrete solutions are used increasingly in both batch and continuous process control.Today's control hardware are constructed from many of the same standard industry components such as Intel processors. Therefore the only real difference between control systems is at the software level.

controller system of industrial automation

Analogue vs Digital Controllers

It is indeed difficult to say that analogue controllers are definitely better than digital controllers. The point is, they both work. Analogue controllers are based on mechanical parts that cause changes to the process via the final control element. Again like final control elements, these moving parts are subjected to wear and tear over time and that causes the response of the process to be somewhat different with time. Analogue controllers control continuously.Digital controllers do not have mechanical moving parts. Instead, they use processors to calculate the output based on the measured values. Since they do not have moving parts, they are not susceptible to deterioration with time. Digital controllers are not continuous. They execute at very high frequencies, usually 2-3 times a second.Analogue controllers should not be confused with pneumatic controllers. Just because a controller is analogue does not mean it is pneumatic. Pneumatic controllers are those that use instrument air to pass measurement and controller signals instead of electronic signals. An analogue controller can use electronic signals. Compared to pneumatic controllers, electronic controllers (can be analogue or digital) have the advantage of not having the same amount of deadtime and lag due to the compressibility of the instrument air.

The element linking the measurement and the final control element is the controller. Before the advent of computers, the controllers are usually single-loop PID controllers. These are manufactured to execute PID control functions. These days, the controllers can do a lot more, however, easily 80 to 90% of the controllers are still PID controllers.

for more information,

free download : http://www.pacontrol.com/download/process-control-systems.pdf